Happy Fibonacci Day Foldscopers! Fibonacci Day is celebrated on November 23rd because of the sequence of numbers in the date when written out (1-1-2-3).

What is the Fibonacci Sequence?

A Fibonacci sequence of numbers is formed when each sequential number is the sum of the two prior numbers. For example:

0, 1, 1 (made from 0+1), 2 (made from 1+1), 3 (made from 1+2), 5 (made from 2+3), etc.

0, 1, 1, 2, 3, 5, 8, 13, 21, 34, 55…

Because 1-1-2-3 are the first four numbers of the Fibonacci sequence, that is the day we celebrate it! Let’s learn more about this series of numbers.

Who discovered this pattern?

Leonardo Pisano was an Italian mathematician from the 12th century who is best known for discovering this numerical pattern of numbers. Fibonacci was his nickname and that is the name that was given to this interesting pattern of numbers.

Why is it interesting? Well, scientists and mathematicians have found this sequence of numbers represented many times in the natural world. If you count leaves, flower petals, or seeds on many plants you will find the numbers in the Fibonacci sequence. It is thought to represent efficiency in placing leaves or petals on plants to provide the optimal spacing and exposure to sunlight or placement of seeds for maximizing packing in a flower head. And the pattern of numbers actually creates a spiral (it is clearly seen in the daisy and oak seed below)!

 

Figures 1a and 1b: A daisy and a seed of an overcup oak showing the Fibonacci sequence spiral. (Photo Credit: Holly A. Stuart)

Models:

An interesting aspect of the Fibonacci sequence is that you can take the list of numbers and make a model showing how it appears in nature. The following figure shows how the numbers can be represented by blocks on graph paper to form a spiral. How big of a Fibonacci spiral can you make?

Figure 2: Model of the Fibonacci spiral (Photo Credit: Holly A. Stuart)

Once you see how the model works, you will start to see the Fibonacci sequence all around you! The Fibonacci spiral can be seen in pine cones, sunflowers, even the inside of a red cabbage!

 

Figure 3a and 3b: The bottom of a pine cone and a cross section of a red cabbage (Photo Credit: Holly A. Stuart)

Pattern Connection:

People are able to easily recognize patterns in the natural world. Finding patterns is an important skill to have because it helps us classify objects, make predictions about future natural phenomena, and provide evidence to explain observations. This ability to identify patterns will help you to start finding the Fibonacci spiral all around you. You may be surprised to see how common this pattern is!

Take a Closer Look at the Fibonacci Sequence

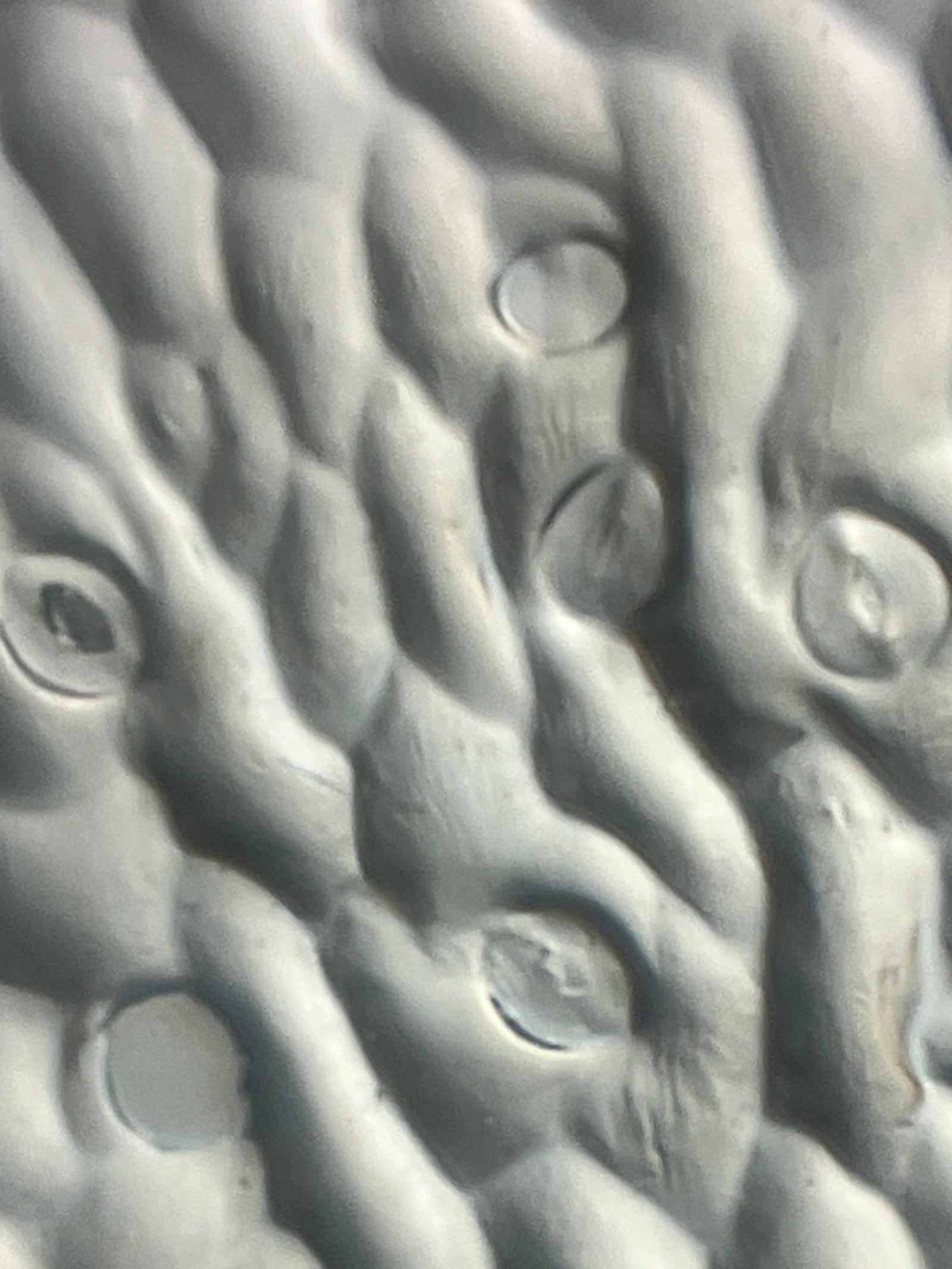
A snail shell is a perfect way to study the Fibonacci spiral up close. If you look at the shell you will see that the spiral is very large on the outside and gets smaller and smaller as you get to the center. But how far does the spiral go? Using a Foldscope will let you observe the spiral at the very center on a microscopic level!

  

Figure 4a and 4b: Snail shell seen with the naked eye and a snail shell viewed with the Foldscope. (Photo Credit: Holly A. Stuart)

What other Fibonacci spirals can you study in a Foldscope?

Have fun discovering the Fibonacci sequence in the world around you and making your own Fibonacci models! Share your microscopic Fibonacci observations on theMicrocosmos and use social media to tag us with your Fibonacci sequence explorations, creations, and discoveries! We love to see how Foldscopers around the world are using their Foldscopes in new and innovative ways!

Facebook: @Foldscope

Twitter: @TeamFoldscope

Instagram: @teamfoldscope

Sources:

https://science.howstuffworks.com/math-concepts/fibonacci-nature.htm

https://www.mathnasium.com/blog/14-interesting-examples-of-the-golden-ratio-in-nature